so sin (11x)cos (6x)cos (11x)sin (6x)=√3/2 sin (11x 6x) = √3/2 sin 5x = √3/2 5x = 60° or 1° x = 15 or 24° the period of sin 5x = 360/5° = 72° you asked for all solutions, which is not possible, since we have an infinite number of solutions, the general solution would beEach of these angles has different values with different trigonometric functions Note 1 cos theta = 1 Cos θ; The delta or mesh connection (Δ) system is also known as the threephase threewire system (3phase 3 wire) and is the most preferred system for AC power transmission when for distribution, a star connection is commonly used In the interconnection system delta (also denoted by Δ), the three phases or the starting end of the coil are

Trigonometry
Cos(x+3/2pi)
Cos(x+3/2pi)-Start studying Unit Circle in Degrees Learn vocabulary, terms, and more with flashcards, games, and other study tools2𝜋 Give your answer in This question hasn't been solved yet Ask an expert Ask an expert Ask an expert done loading A) Find all degree solutions for the following (Enter your answers as a commaseparated list



Www Uplifteducation Org Cms Lib Tx Centricity Domain 291 Review 3 key Pdf
Principal solution of cos x = √3/2 1 See answer plus Add answer 5 pts report flag outlined bell outlined Log in to add comment hsilodiya2784 is waiting for your help Trigonometric Ratios Of Some Specific Angles With Examples (ii) 4cot 2 45º – sec 2 60º sin 2 60º cos 2 90º Sol (i) When x = 30°, we have 2x = 60° (ii) When x = 30°, we have 2x = 60° ⇒ 3θ = 60° ⇒ θ = ° Example 7 If θ is an acute angle and sin θ = cos θ, find the value of 2 tan 2 θ sin 2 θ – 1 Example 8Click here👆to get an answer to your question ️ Write the value of the expression tan ( sin^1x cos^1x2 ) , where x = √(3)2
This preview shows page 95 99 out of 141 pages Since A = 1 and B = − 1, k = radicalbig 1 2 (− 1) 2 = √ 2 Then we want a φ so that cos φ = 1 √ 2 and sin φ = − 1 √ 2 The reference angle is then, ref = cos − 1 parenleftbigg 1 √ 2 parenrightbigg = sin − 1 parenleftbigg 1 √ 2 parenrightbigg = π 4 Since cos φ > 0 and sin φ < 0 we know φ is in Quadrant IV, so φClick here👆to get an answer to your question ️ Find the principal solution of the following equations sin x = √(3)2 Join / Login > 11th > Maths > Trigonometric Functions > Trigonometric Equations The maximum value of the expression sin 2 θ 3 sin θ cos θ 5 cos 2We can solve this using two methods 1 From log book or log table of cos inverse 2 Using scientific calculator To use method 1 first convert the cos into cos^(1){cos inverse} so Cos(x) = So cos^(1) ()=x Find the near value of x
Trigonometric Functions are formed when trigonometric ratios are studied in terms of radian measure for any angle (0, 30, 90, 180, 270)These are also defined in terms of sine and cosine functions In this article, we will provide you with all the details on trigonometric functions such as value in degree, radians, complete trigonometric table and other relevant information Write the value of the expression tan ((sin^1 x cos^1 x)/2), when x = √3/2 asked Apr 6 in Trigonometry by Takshii ( 346k points) inverse trigonometric functionsRD Sharma Solutions for Class 12science Mathematics CBSE, 4 Inverse Trigonometric Functions All the solutions of Inverse Trigonometric Functions Mathematics explained in detail by experts to help students prepare for their CBSE exams




Find The Solution Of Sinx Sqrt 3 2



Unit Circle Calculator Inch Calculator
cos (x) = √3/2 cos (x) = cos(5π/6) The genaral solution of cos(θ) = cos(α) is θ = 2nπ ± α, where n is an integer ⇒ x = 2nπ ± 5π/6 If n = 0, x = 2(0)π (5π/6) and x = 2(0)π (5π/6) = 5π/6 and 5π/6 If n = 1, x = 2(1)π (5π/6) and x = 2(1)π (5π/6) = 2π 5π/6 and 2π 5π/6 = 17π/6 and 7π/6,Pdfs can be downloaded easily from the links provided below Chapter 5 Trigonometric Functions contains three exercises and the RD Sharma Solutions present in this page provide solutions to the questions present in each exercise Now, let us have a look at the concepts discussed in this chapter Trigonometric functions of a real numberScan with Trigonometric equations sin(x) = cos(x)cos(3x−π)·tan 3x− π 4 ˚ =0 tan(x)=√ 3 3 sinx− √ 3cosx =0 sin2(x)−3sin(x)2=0 sin(2(x45 )) = 1tan(x)∗sin˜π 2 −x ˚ =0 2tan3(x)=tan(x) sin(x)cos(2x)cos(x)sin(2x)=√ 3 2 Irrational equations √ x =9 x−2 3x =5 ˝ 2x √



Limit Trigonometric Function Sin X Pi 6 Sqrt 3 2cosx Youtube




Find All Solutions Of The Equation In The Interval Chegg Com
Exams Banking Entrance Exams Defence Exams Engineering Exams Finance Entrance Exams GATE Exams Insurance Exams International ExamsC) Solve the equation for x if 0 ≤ x < The dissimilarity between the two vectors 'x' and 'y' is given by – ∴ Dis (x, y) = 1 Cos (x, y) = 1 049 = 051 The cosine similarity between two vectors is measured in 'θ' If θ = 0°, the 'x' and 'y' vectors overlap, thus proving they are similar If



Find The Integral Int Limits 0 Pi 6 Sin X Cos 2 X Mathrm Dx Mathematics Stack Exchange



3
Wnte the Value of the Expression Tan ( Sin − 1 X Cos − 1 X 2 ) , When X = √ 3 2 Department of PreUniversity Education, Karnataka PUC Karnataka Science Class 12 Textbook Solutions Important Solutions 984 Question Bank Solutions 100 Concept Notes & VideosWhat is the principal value of cos−1(−√32) ?(a) 2 sin(x) 1 = 0 (b) 2 cos(x) √ 3 = 0 (c) tan(x) = √ 3 2 Use the triangle below to find all solutions of the equations below Give exact answers in radians 1 2 radicalbig 2 √ 2 1 2 radicalbig 2 − √ 2 1 π/ 8 3 π 8 (a) sin(x) = 1 2 radicalBig 2 √ 2 (b) cos(x) = − 1




What Is The Solution Of Math Cos X Sqrt 3 Math Quora




Solving Trig Equation Of Cos X 3 2 Youtube
Examples Based on Signs of Trigonometric Ratios Example – 01 State the signs of following Trigonometric Ratios (Functions) sin 675 o sin 675 o = sin (360 o 315 o) = sin (315 o) = sin (270 o 45 o) Thus the angle 675 o lies in the fourth quadrant, where sin function is negative Hence sin 675 o is negative sin 159 o sin 159 o = sin (90 o 69 o) Thus the angle 159 o lies in If 2y = (cos1 (√3 cos x sin x /cos x √ 3 sin x))2 , x ∈(0, π/2) then dy/dx is equal to (1) x π/6 (2) π/3 x (3) π/6 x (4) 2x π/3Cos x = 0 ⇔ x = π/2 kπ, k ∈ Z cos x = 1 ⇔ x = 2kπ, k ∈ Z cos x = 1⇔ x = π 2kπ, k ∈ Z Example Solve 2cos x – √3 = 0 Solution Let us rewrite the equation in terms of cos x Then we get cos x = √3/2, and we know arccos √3/2 = 30° = π/6



1



Sin X Square Root 2 2 X Pi 3 Cos X 1 2 X Chegg Com
Show that 12 cos 30° 2 tan 60° can be written in the form√ k where k is an integer Firstly work out (using the sin cos tan triangle and soh cah toa) what cos 30° and tan 60° are equal to so tan 60° = √3 and cos 30° = √3 / 2 then substitute these values into the euqation giving 12 x √3 / 2 2 √3 which can be simplified to 6Cos x = √3/2 Solution cos x = √3/2 > 0 Principal value of x must be in 0, π Since cos x is positive the principal value is in the first quadrant We have to think about the angle of cos for which we get the value √3/2 cos π/6 = √3/2 and π/6 ∈ 0, π cos x = √3/2 And this happens at x = pi/6 and 11pi /6 So the solutions are x = 0 , x = pi/6 and x = 11pi/6 CPhill




Cos2x Cosxu003d0



Exact Trig Values
Transcript Example 18 Find the principal solutions of the equation sin x = √3/2 sin x = √3/2 We know that sin 60° = √3/2 Here sin is positive, sin is positive in Ist and IInd quadrant Value in Ist quadrant = 60° Value in llnd Quadrant = 180 – 60 = 1° So, principal solution are x = 60° and 1° x = 60/180 𝜋 and 1/180 𝜋 x = 𝜋/3 and 2/3 πAnswer (b) 300 Explanation In this example, we can find the value of θ by putting values given in options It is the hit and trial approach The value that satisfies the given equation will be considered as the value of θ Given 4 cos 2 θ 4√3 cos θ 3 = 0 So, in option A θ = 60 0 is given, let's put it and see whether the equation will be satisfied or notHi interval 0 2pi) sin^2(x)√3sin(x)1= cos^2(x) (1cos^2(x) √3sin(x)1= cos^2(x) √3sin(x)= 2(cos^2(x) 1) √3 sin(x)= 2sin^2(x)



What Is The Limit Of Cos 3 X 3 Cos X P 2 X When X P 2 Quora



1
Eg 1 cos 30° = 1 Cos 30° = 1 x √3/2 = √3/2 Also, sin (90 θ) = cos θ and Cos (90 θ) = sin θ Also remember sin 45 = cos 45 = 1/√2 The value of sin θ and cosCos𝑥− ½ sin x sin x = √3 2 cos𝑥 1 2 sin𝑥 M1 = cos x cos30 sin x sin30 M1 = cos (x – 30)o (RHS) M1 Trignometry Solving Trig Equations 1 Solve the equation sec x = 5, giving all the values of x in the interval 0 ≤𝑥 ≤2𝜋 in radians to two decimal places (3) b15π cos x < √3/2 correspond à l'angle remarquale cos π/6 = √3/2 c a π/6 15π 0 12π Les symétries géométriques permettent d'en déduire les trajets pour déterminer b les valeurs exactes des bornes à partir de la valeur exate de l'angle remarquale




Reshite Trigonometr Uravnenie Cos X Kornya Iz 3 Delennaya Na 2
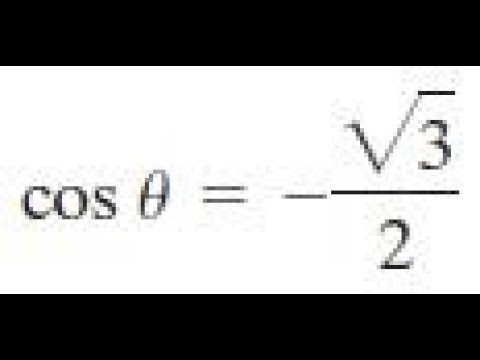



Cos X Sqrt 3 2 Give The General Formula Solutions Youtube
Sin2 x cos2 x = 1 Example sin x = √3/2 Answer (√3/2)2 cos2 x= 1 ¾ cos 2 x = 1 cos 2 x = 1 – ¾ √cos 2 x = (√1/4) cos x = ½ These Pythagorean Identities are also included tan2 x 1 = sec2 x 1 cot2 x = csc2 x Establishing Other Identities • To verify an identity equals to the other, many steps are taken(Enter your answers as a commaseparated list) cos 2x cos x − sin 2x sin x = √3/2 X=?Cos x = 0 ⇔ x = π/2 kπ, k ∈ Z cos x = 1 ⇔ x = 2kπ, k ∈ Z cos x = 1⇔ x = π 2kπ, k ∈ Z Example Solve 2cos x – √3 = 0 Solution Let us rewrite the equation in terms of cos x Then we get cos x = √3/2, and we know arccos √3/2 = 30° = π/6




Tanx 4 3 X In Quadrant Ii Find The Value Of Sinx 2 Cosx 2 Tanx 2



Find The Simplified Form Of Cos 1 3 5 Cos X 4 5 Sin X X 3p 4 P 4 Studyrankersonline
5 Find the complete exact solution of sin x = √3/2 9 Solve cos 2x – 3sin x cos 2x = 0 for the principal value(s) to two decimal places 21 Solve tan^2x tan x – 1 = 0 147,610 results, page 14If you mean cos (90° x) = √3 / 2 then you must write the degree sign Then we have cos (90° x) = cos 30° So 90° x = ±30° k360° for any integer k , x 90° = ±30° k360° for any integer k (changed "±" and k) x = 60° k360° or 1° k3Cos x = √‾3/2 , Matematika 3 http//wwwmimsragacom/ zbirka potpuno riješenih zadataka
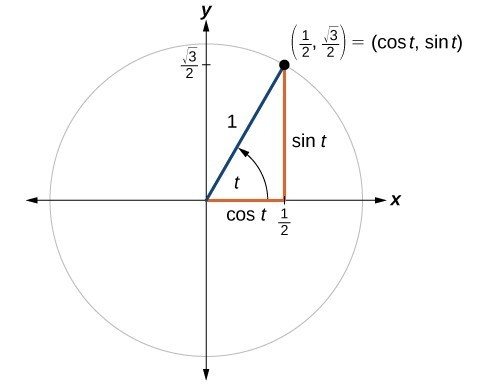



Unit Circle Sine And Cosine Functions Precalculus Ii



If Cos X Then What Is Tan X Equal To Quora
Driving Tests (India) Mass Communication and Journalism NCHMCT JEE Previous Papers Online NTSE Previous Papers Online NIFT UG & PG Previous Papers Online Postal Exams Model Papers Police Exams AP Police and SI Exam Tests Bihar Police Exam TestsYou can put this solution on YOUR website!Answer to Given f(x) = cos(x) and x =π/6T2(x)= (√3/2)(1/2)(x(π/6))(1/4)√3(x(π/6)2)Let a be a positive real number and l




How Do You Find The Equation To The Tangent Line Of Y Cos3x And Its Tangent At The Point Pi 4 Sqrt2 2 Socratic




Lt X Tends To Pi 6 2 Sqrt 3 Cosx Sinx 6x Pi 2 Brainly In
(ii) cos −1 √3/2 Solution x = cos −1 √3/2 cos x = √3/2 cos x = cos π/6 x = π/6, where x ∊ 0, π (iii) cosec −1 (1) Solution x = cosec −1 (1) Range of cosec1 x is π/2, π/2, so the required angle lies in the above interval cosec x = cosec (π/2) x = π/2, where x ∊ π/2, π/2 (iv) sec −1 (√2)Trigonometric values of ratios like sine, cos, tan, cosec, cot, and secant are very useful while solving and dealing with problems related to the measurement of length and angles of a rightangled triangle 0°, 30°, 45°, 60°, and 90° are the commonly used values of the trigonometric function to solve trigonometric problemsCos x < √3/2 dans 12π ;




Reshite Uravnenie Cos X 3 2 Shkolnye Znaniya Com



Important Angles In Trigonometry
cos (x) = √3/2 cos (x) = cos(5π/6) The genaral solution of cos(θ) = cos(α) is θ = 2nπ ± α, where n is an integer ⇒ x = 2nπ ± 5π/6 If n = 0, x = 2(0)π (5π/6) and x = 2(0)π (5π/6) = 5π/6 and 5π/6 If n = 1, x = 2(1)π (5π/6) and x = 2(1)π (5π/6) = 2π 5π/6 and 2π 5π/6 = 17π/6 and 7π/6, where, p = normal distance from the origin & ω = angle which makes by the normal with positive xaxis Here p = 5 & ω = 30° Putting values x cos ω y sin ω = p x cos 30° y sin 30° = 5 x √3/2 y 1/2 = 5 (√3 𝑥 𝑦)/2 = 5 √𝟑 𝒙 y = 10 Thus, equation of line is √3 𝑥 y = 10 Show MoreSec x = 1/cos x = 1/(1/2) = 2 ∴ sin x = √3/2, tan x = √3, cosec x = 2/√3, cot x = 1/√3 sec x = 2 (iii) tan x = 3/4, x in quadrant III In third quadrant, tan x and cot x are positive sin x, cos x, sec x, cosec x are negative By using the formulas, sin x = √(1 – cos




Solve The Trigonometric Equation Sqrt 3 Cos X Sin X 1 Youtube



How To Find The Value Of X From 3sinx Cosx Quora
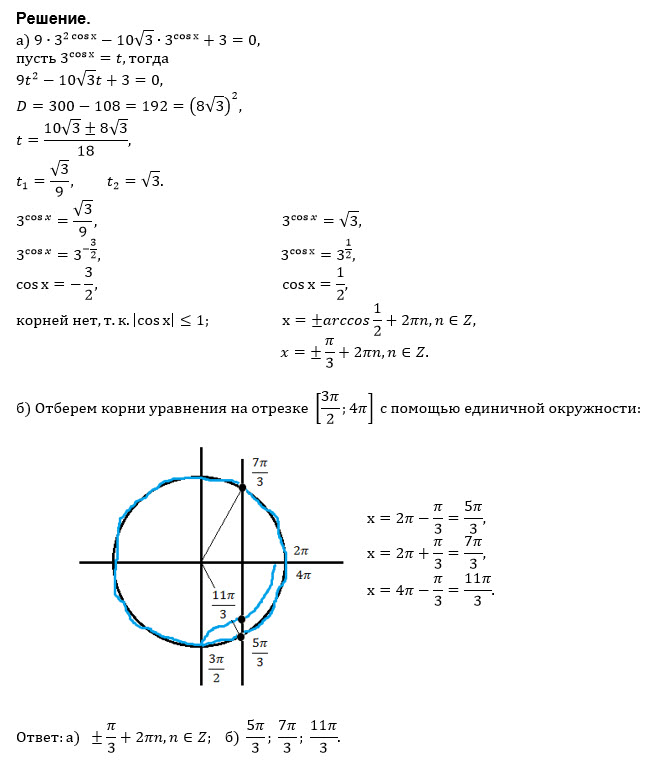



A Reshite Uravnenie 9 3 2cosx 10sqrt 3 3 Cosx 3 0 B Ukazhite Korni Etogo Uravneniya Prinadlezhashie Otrezku 3pi 2 4pi




Find The Solution Of Sinx Sqrt 3 2




Trigonometry Solve 2 Cos X Sqrt 2 0 Youtube




Solve Sin X 30 2 Cos X 45 0 360 Trigonometric Equation Youtube



Graph Domain And Range Of Arccos X Function



Www Drfrostmaths Com Getfile Php Fid 479
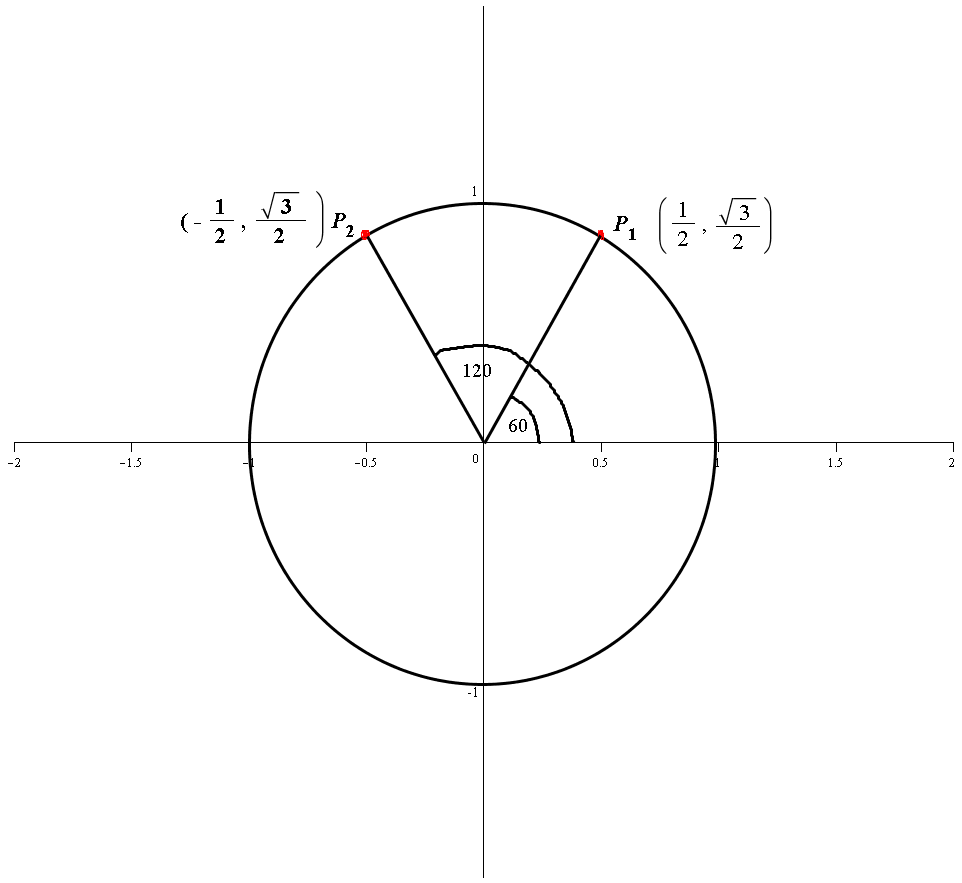



If The Point X Sqrt 3 2 Is On The Unit Circle What Is X Socratic
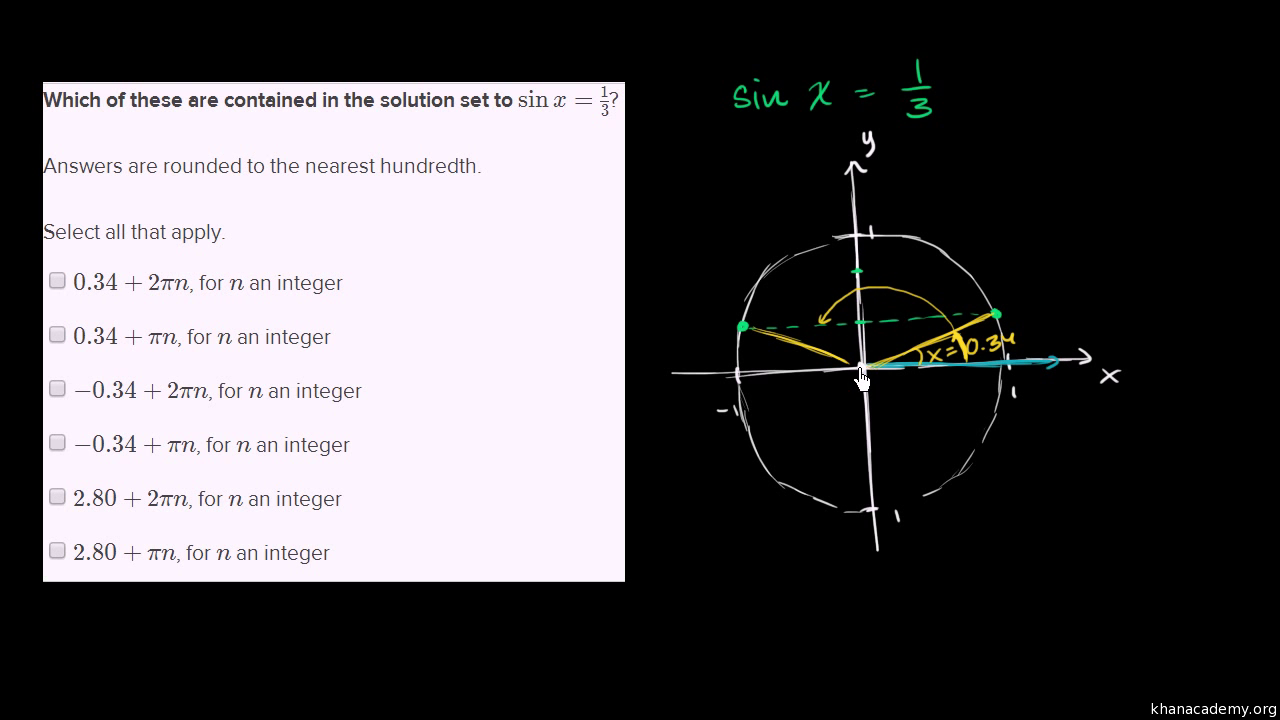



Solving Sinusoidal Equations Of The Form Sin X D Video Khan Academy




Prove That Sin 1 1 2 Cos 1 Sqrt3 2 Cos 1 1 2 Mathematics And Statistics Shaalaa Com




Solution Find The Exact Value Of The Trigonometric Function At The Given Real Number A Cos 11 Amp 960 6 B Cos Amp 8722 11 Amp 960 6 C Cos 17 Amp 960 6 Please Exp




How Do You Graph F X Sinx Sqrt 3 Cos X For X Is Between 0 2pi Socratic




If Cos X 1 3 And X Lies In Quadrant Iii Find The V



If 0 X Y P And Cosx Cosy Cos X Y 3 2 Then Sinx Cosy Is Equal To Sarthaks Econnect Largest Online Education Community




Cosx 3 2 Ucheba I Nauka



Signs Of Trigonometric Ratios In Diffrent Quadrants Formed Due To Axes




If X Lies In The First Quadrant And Cos X 817 Then Prove That Cos Pi6 X Cos Pi4 X Cos 2pi3 X 3 12 1 2 2317
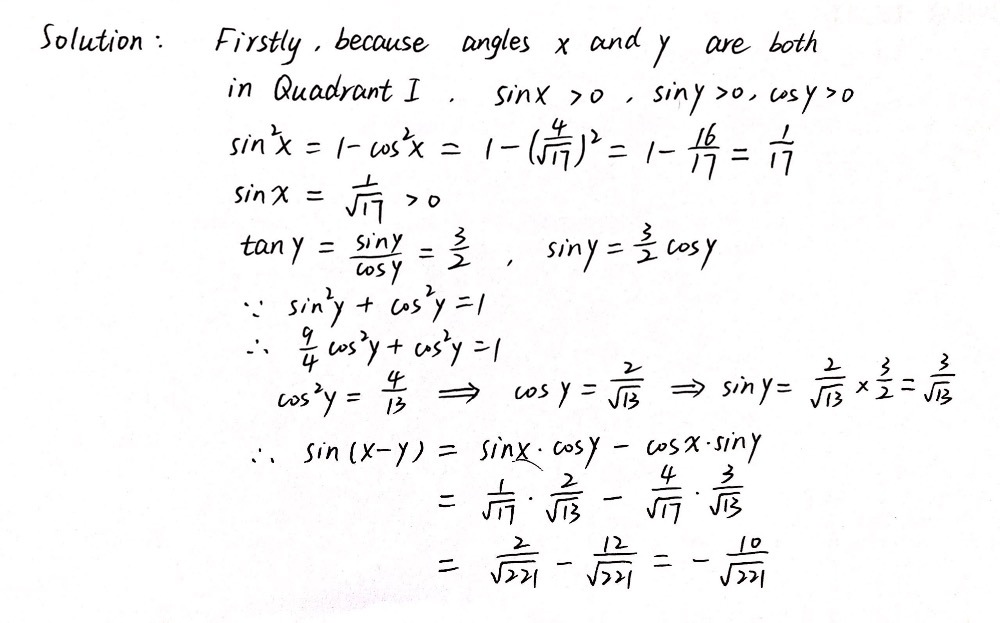



Given That Cos X Frac 4 Square Root Of 17 And Tan Gauthmath




Find All Solutions Of The Equation In The Interval Chegg Com
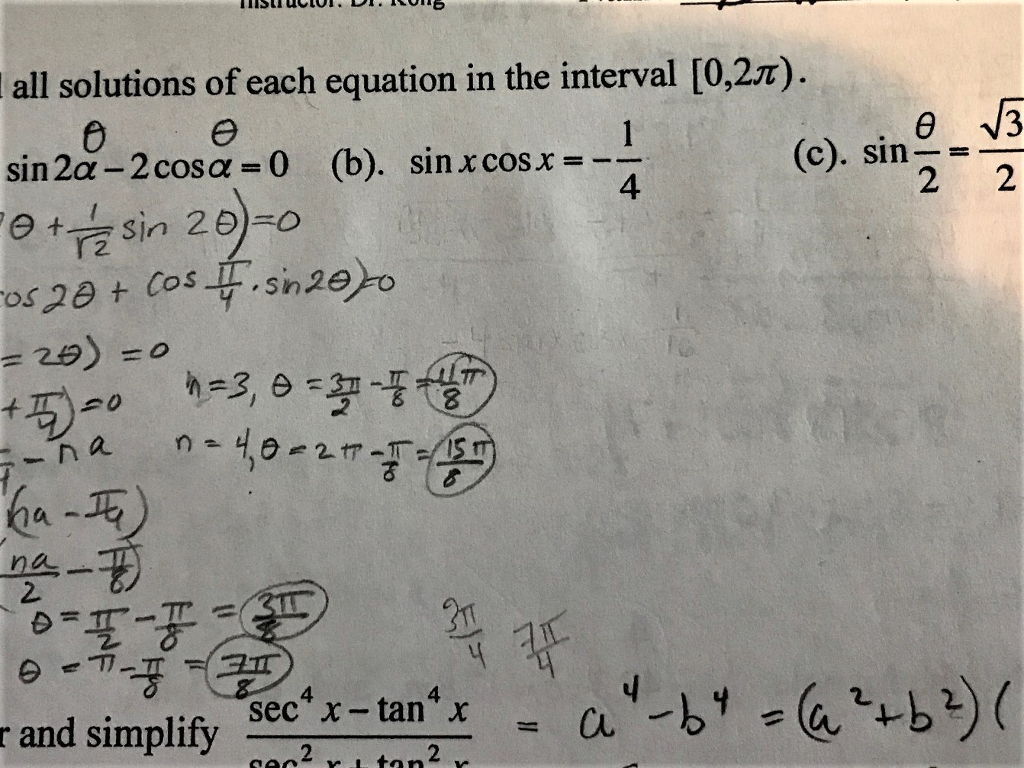



Find All Solutions Of Each Equation In The Interval Chegg Com
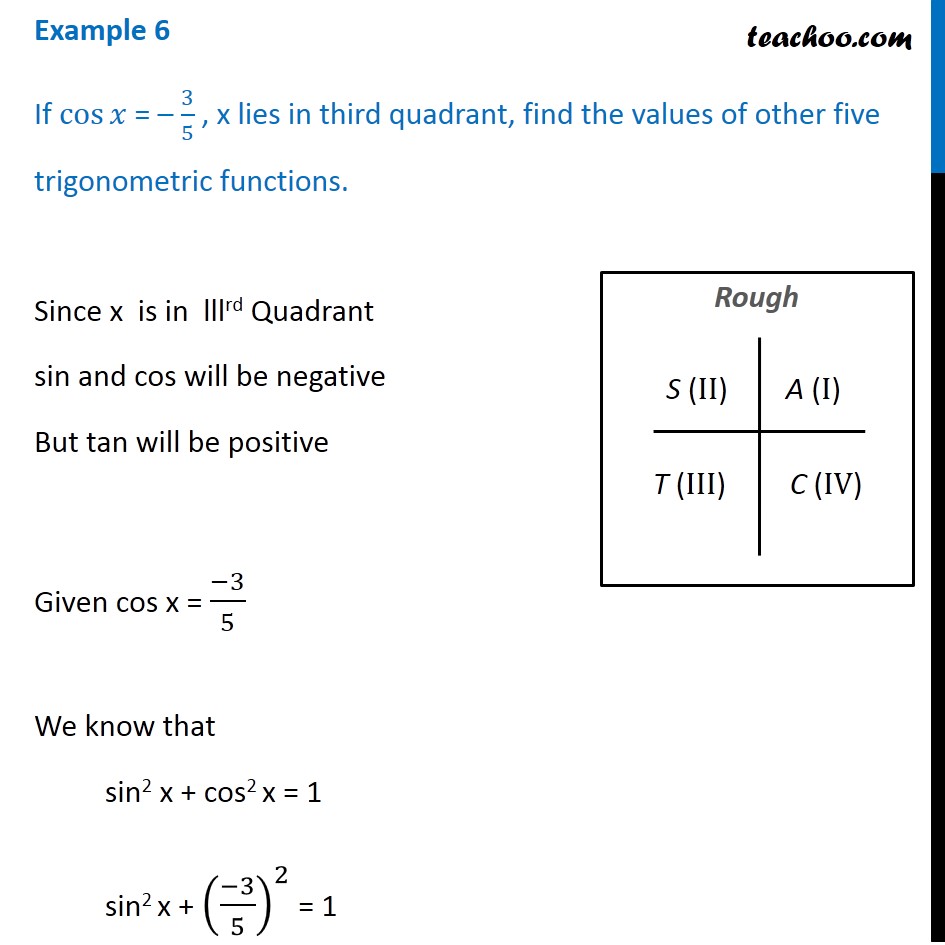



Example 6 If Cos X 3 5 X Lies In Third Quadrant Find



How To Solve A Trigonometric Equation When Cosx Sqrt 3 2 Part 5 Of 8 Youtube




2sinxcosx Formula Gamers Smart



How Do You Find The Critical Points Of Y X 2sinx On The Interval 0 Pi 2 Socratic
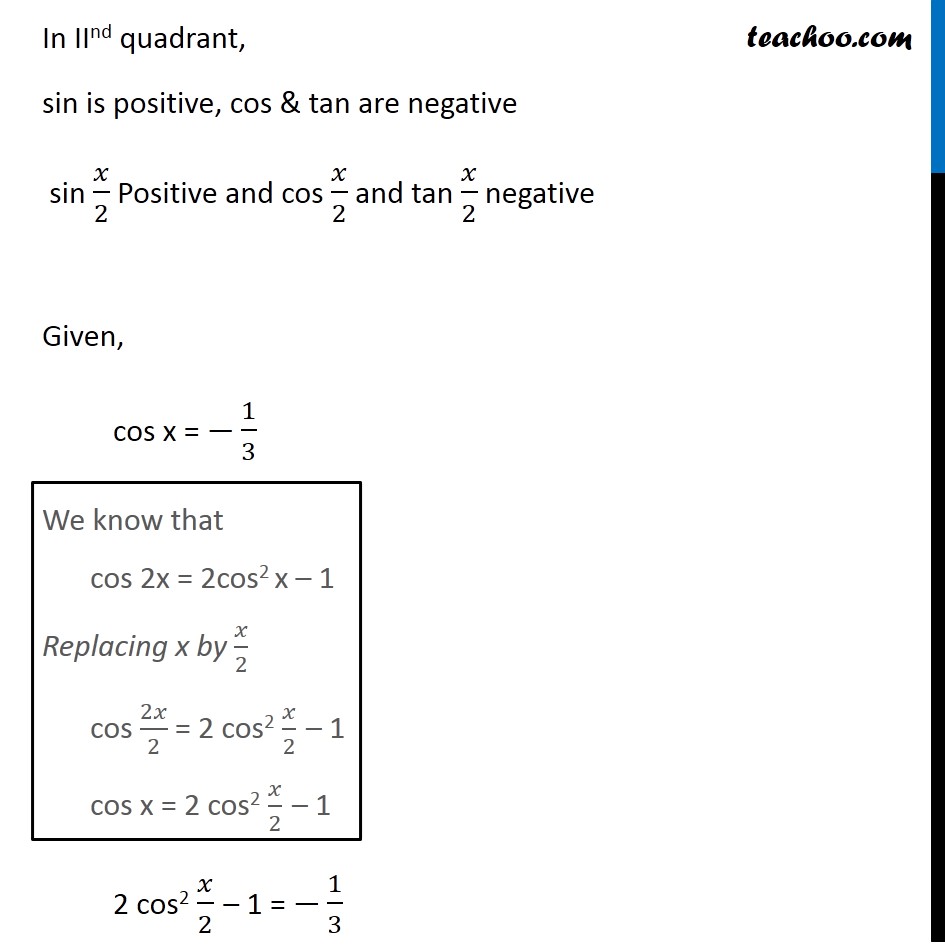



Misc 9 Cos X 1 3 Find Sin X 2 Cos X 2 And Tan X 2




If Cos A B 3 5 And Tanatanb 2 Then
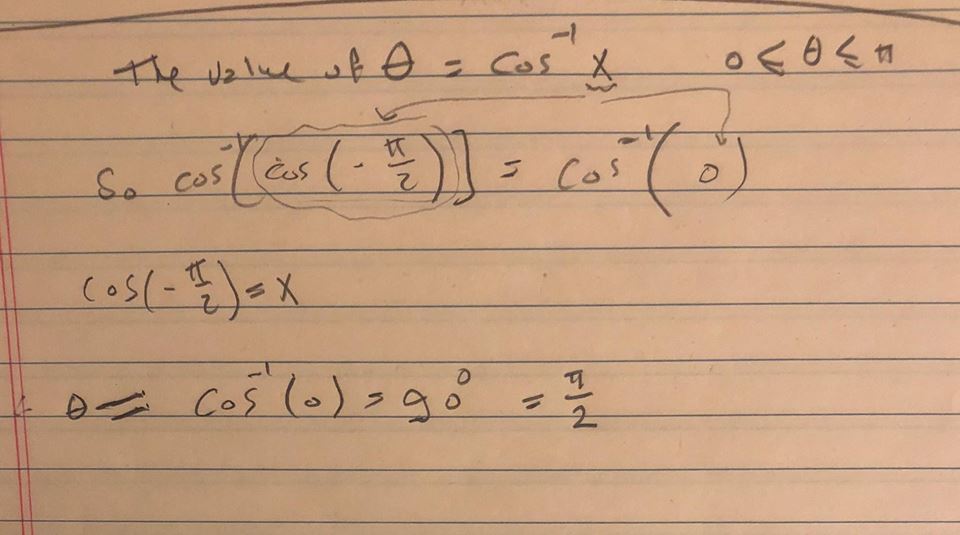



How Do You Evaluate Cos 1 Cos Pi 2 Socratic




Find General Solution 3 1 Cos X 3 1 Sin X 2 Brainly In




How Do They Know The Max Value Of Square Root Of 1 Cosx On 0 1 Is Equal To Square Root Of 2 Calculus



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap18 Calculus Ab Q5 Pdf




Reshit Uravnenie Cos X 2 2 Cos X 3 4 Cos 4x 1 2cos X 3 3 Cos X 3 2 Cos X 0 3 Shkolnye Znaniya Com




Solve The Trigonometric Equation Sqrt 3 Cos X Sin X 1




Prove That Sin X P 6 Cos X P 3 3sinx Brainly In
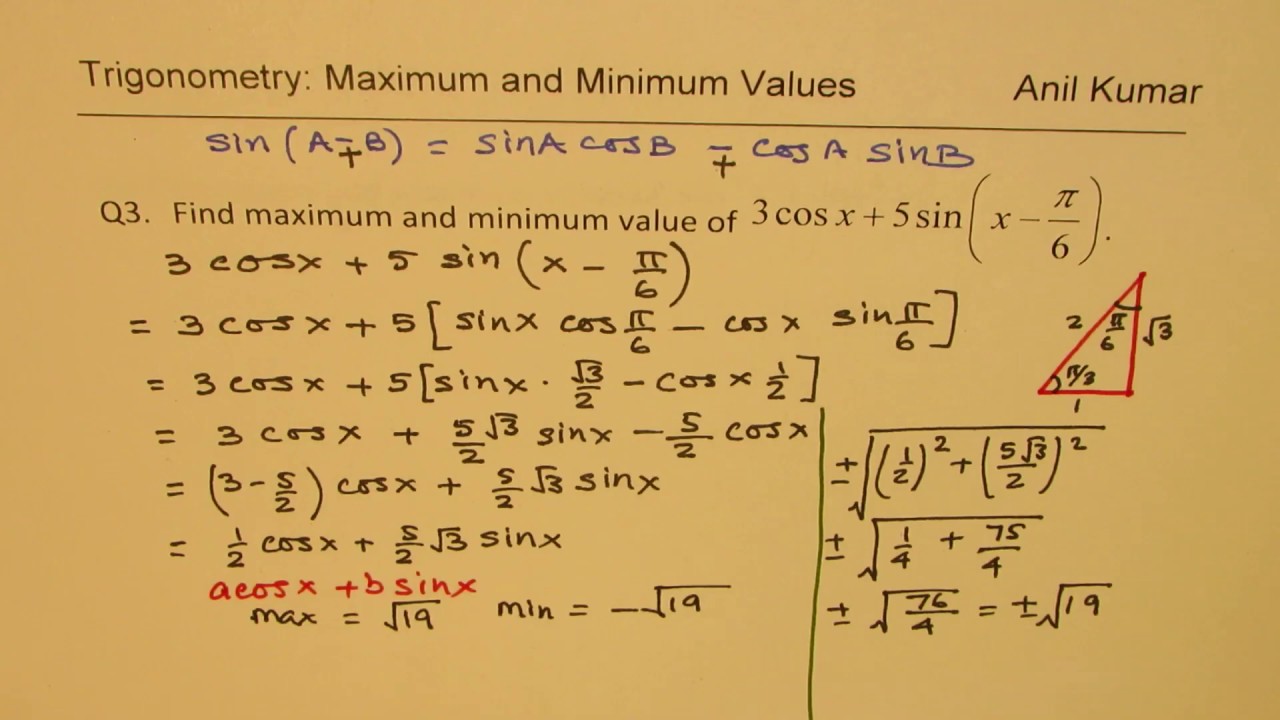



Maximum Minimum For Trigonometric Functions 3 Cos X 5 Sin X Pi 6 Ssc Cgs Test Youtube
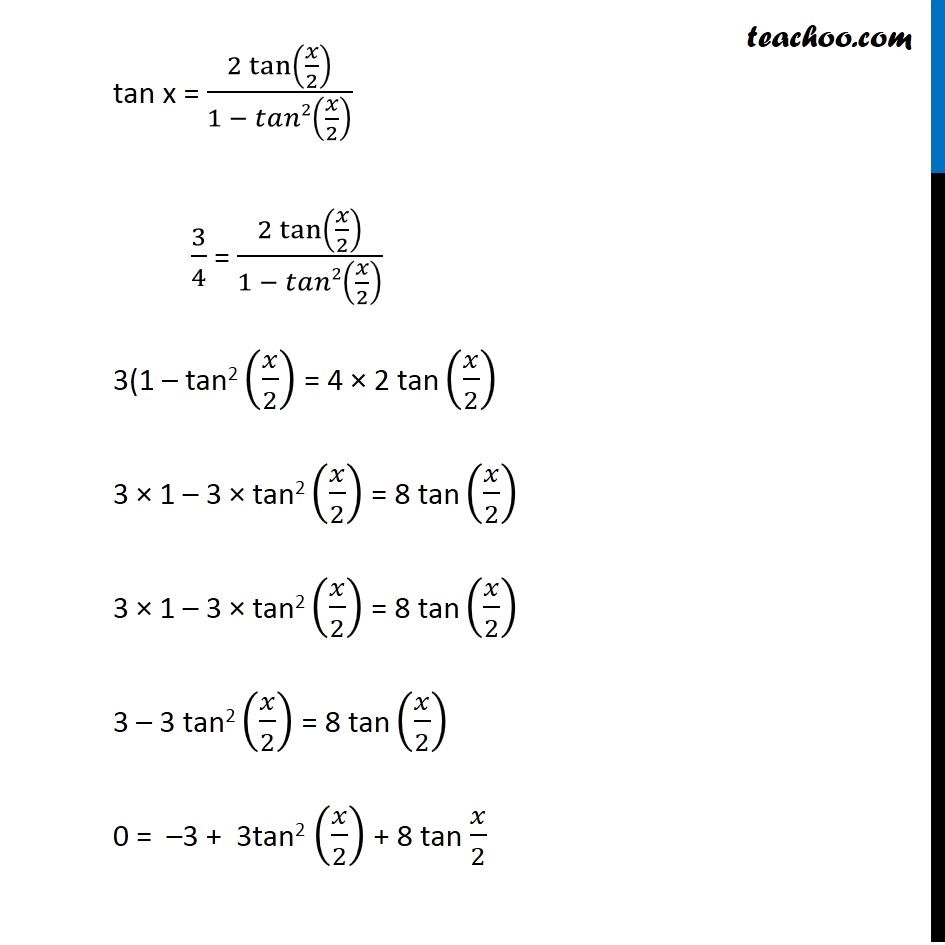



Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2



Www Uplifteducation Org Cms Lib Tx Centricity Domain 291 Review 3 key Pdf




The Principal Solution Cosx Sqrt 3 2 Is



What Is The Principal Value Of Cos 1 3 2 Sarthaks Econnect Largest Online Education Community




Expressing In The Form A Sin X C Mathematics Stack Exchange
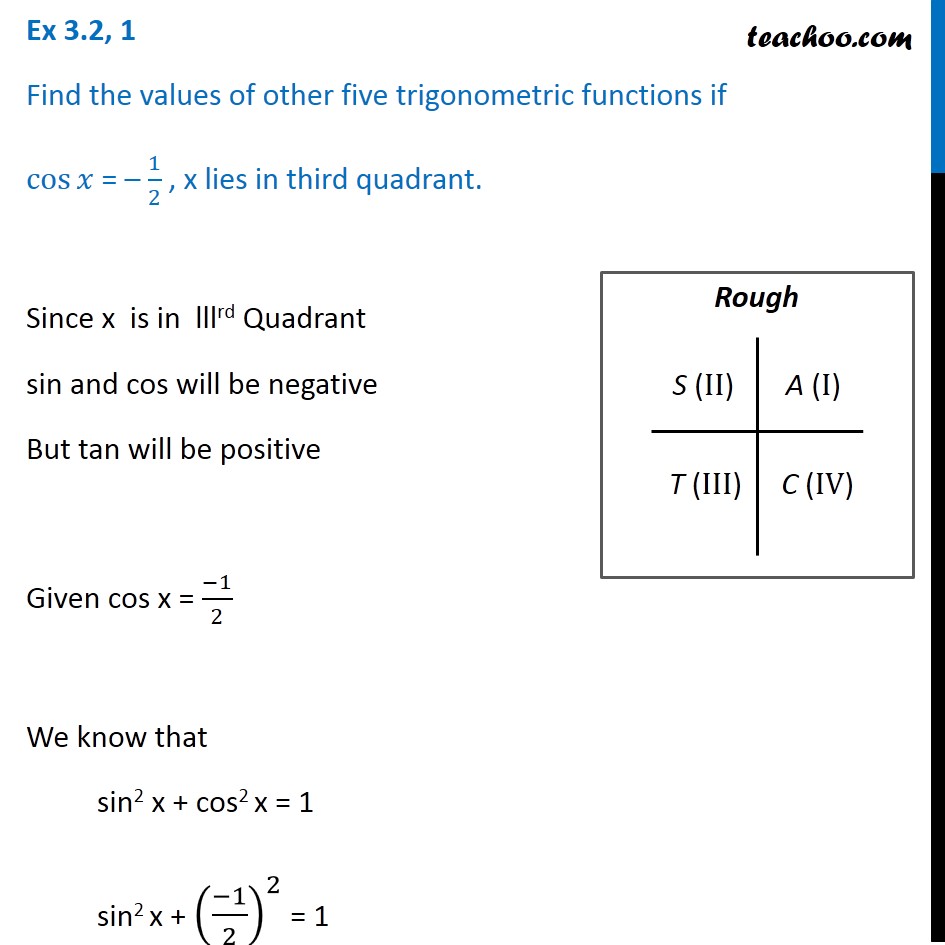



Ex 3 2 1 Find Values Of Other Five Trigonometric Functions If Cos X



Given That Tan X 5 2 Where X Is An Obtuse Angle How Do You Find The Value Of Sin X 2 Without Using A Calculator Quora




Find All Solutions Of The Equation In The Interval Chegg Com




Prove That I Cos Pi 3 X 1 2 Cos
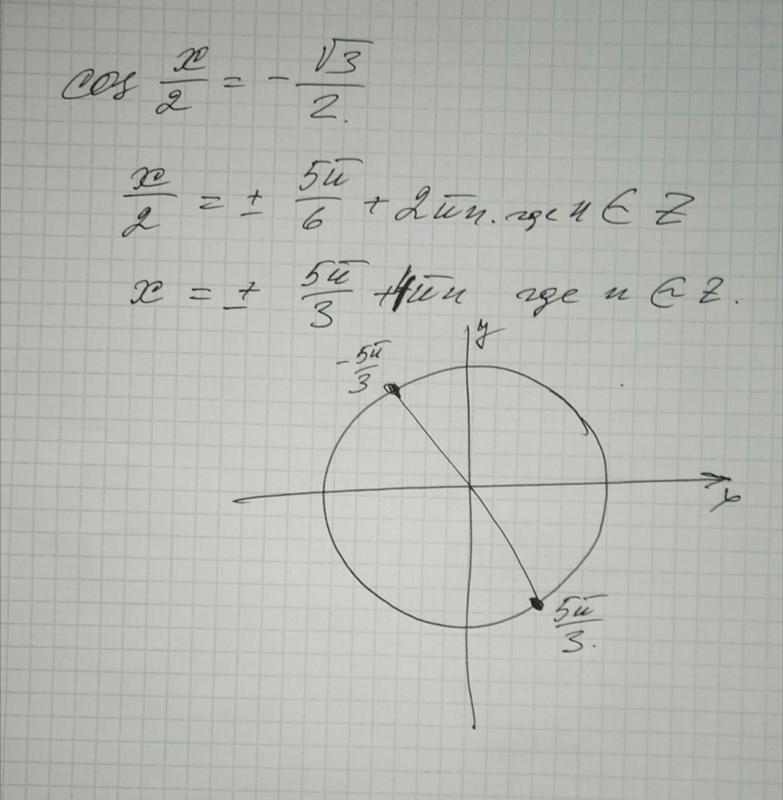



Otvety Mail Ru Cos X 2 3 2 Pozhalujsta Pomogite S Uravneniem Esli Mozhno S Okruzhnostyu



What Is The Solution To Sinx Cosx 3 2 Quora




2sinxcosx2 Cos X Answer Gamers Smart



Maclaurin Series Of Sin X Video Khan Academy




Trig Graphs And Equations Ppt Download



If Sin X Sin Y 1 2 And Cos X Cos Y 3 2 Where X Y 0 P 2 Then Tan X Y Sarthaks Econnect Largest Online Education Community



If Tanx 3 4 P X 3p 2 Find The Value Of Sin X 2 Cos X 2 And Tan X 2 Sarthaks Econnect Largest Online Education Community



Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2



Biomath Trigonometric Functions




If 2sin 2x 3cosx Find X Brainly In




13 39 1 If Sin X 3 4 Then Cos X A 2 3 Gauthmath



1




Cos 2 Theta Square Root 3 2 Cos 2 Theta Chegg Com




Reshite Neravenstvo Cos X 3 2 Shkolnye Znaniya Com




Unit Circle



Www Drfrostmaths Com Getfile Php Fid 479



The Other Trigonometric Functions Precalculus Ii




Transforming Acos X Bsin X Into Rcos X A Shortmath
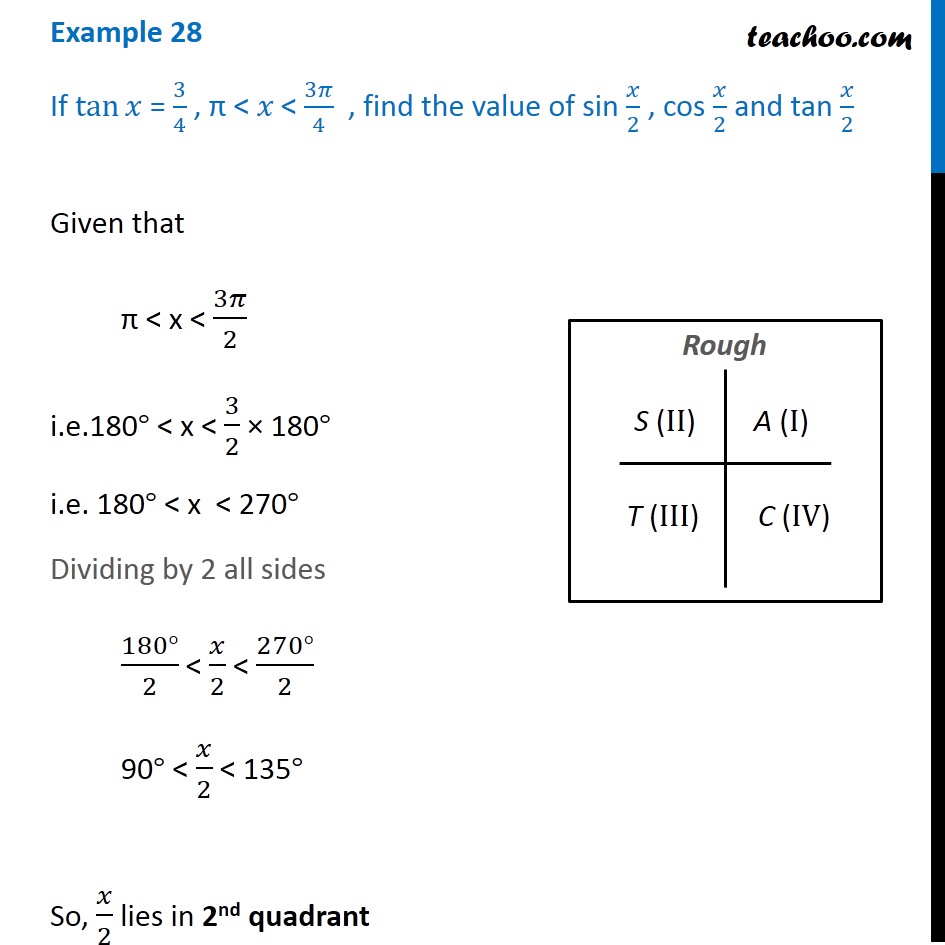



Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2



Curvature And Radius Of Curvature Page 2



How To Calculate The Limit Of Math Frac 2 Sqrt 3 Cos X Sin X 6x Pi Math When Math X Rightarrow Frac Pi 6 Math Quora




Trigonometry
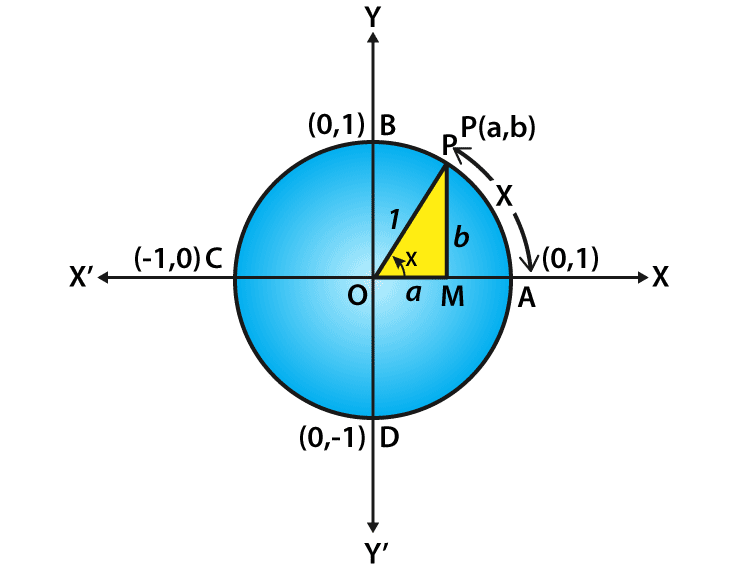



Cos 0 How To Find The Value Of Cos 0




Solve The Equation Sin X 3 Cos X 2 And X Epsilon 30 0 0 0




If 4 Cos 2theta Sqrt 3 2 Sqrt 3 1 Costheta Then Theta Is



Solve The Trigonometric Equation Cos 3x Sin X Sqrt 3 Cos X Sin 3x Mathematics Stack Exchange



Find The Principal Solution Of The Following Equations I Sinx 3 2 Ii Tanx 1 3 Sarthaks Econnect Largest Online Education Community




Solve The Trignometric Equation 2 Sin 3x Pi 4 Sqrt 1 8 Sin2x Cos 2x Mathematics Stack Exchange



Solve Cos X Sqrt 3 2 Youtube
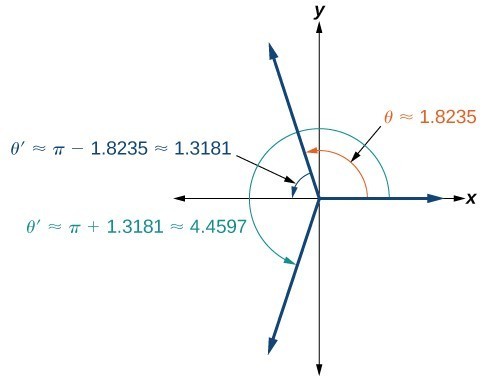



Solving Trigonometric Equations Precalculus Ii




These Statements About Analytic Trigonometry Have Been Proved Shortmath
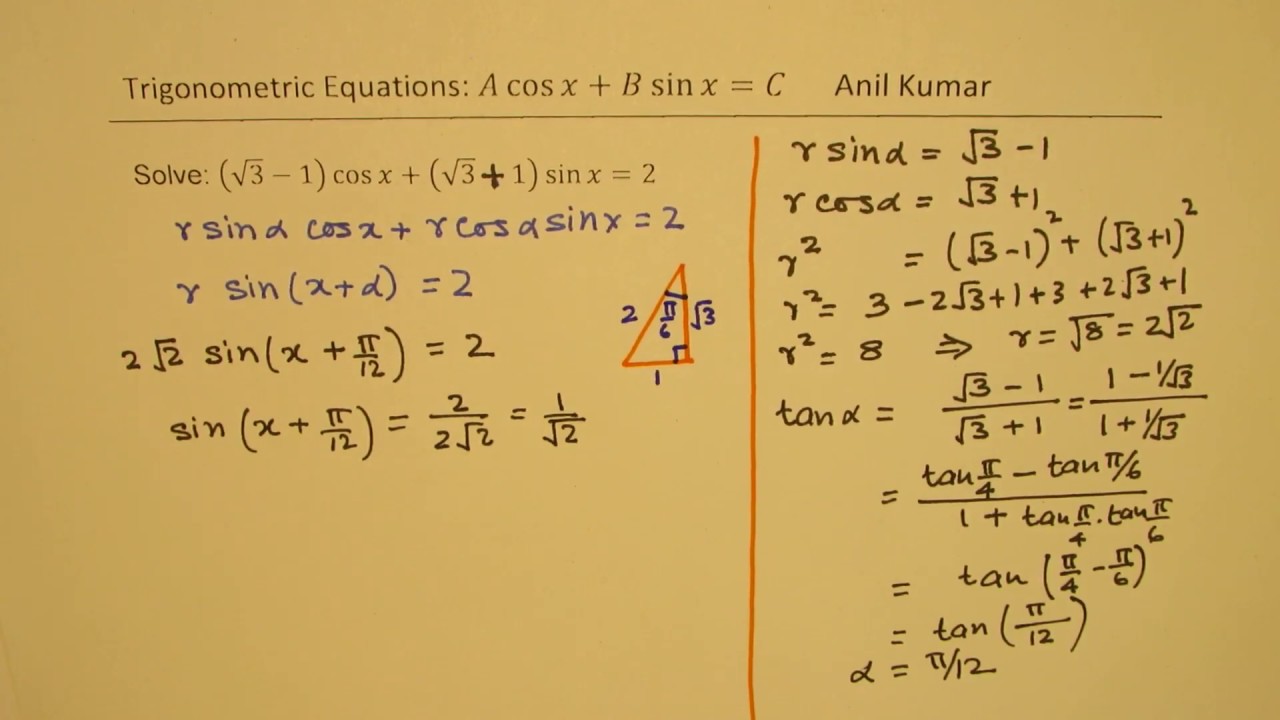



Solve Trigonometric Equation 3 1 Cos X 3 1 Sin X 2 Youtube
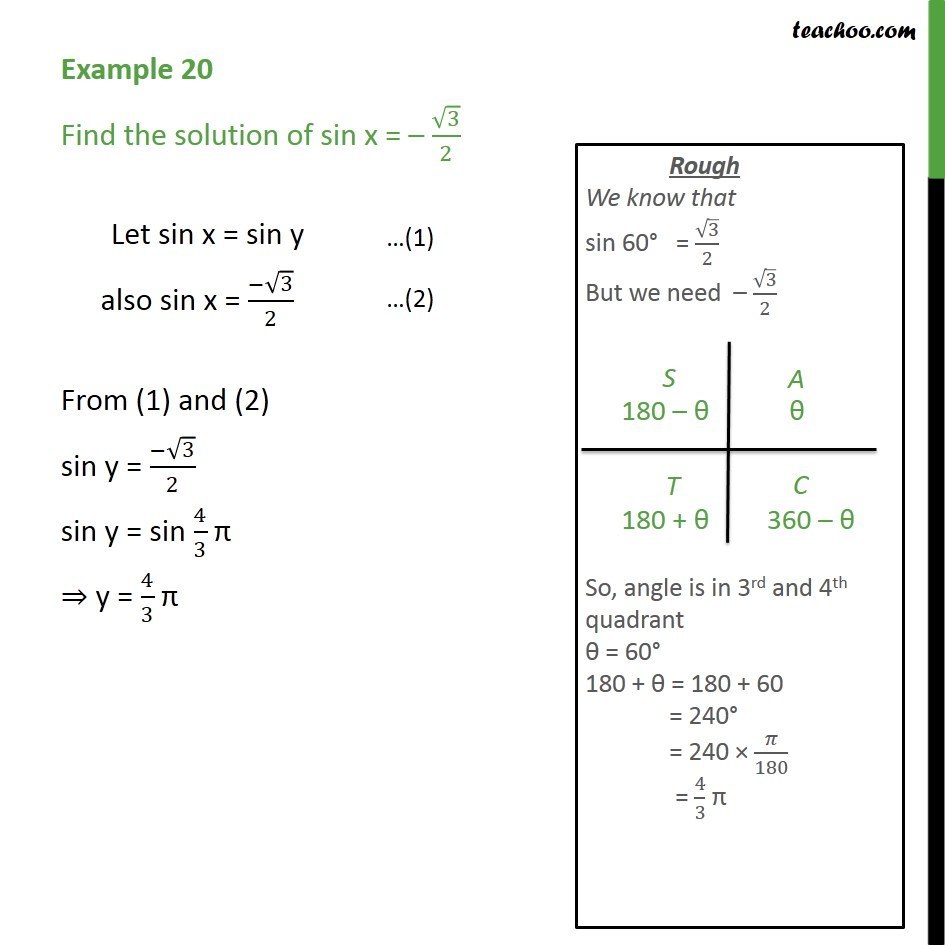



Example Find Solution Of Sin X Root 3 2 Class 11




How Do You Simplify Sinx 1 Cosx 1 Cosx Sinx Socratic




Cos X Sin X 2 3 Find Sin 2x Double Angle Formula Youtube




Solve The Following Equations On The Interval 0 Chegg Com



0 件のコメント:
コメントを投稿